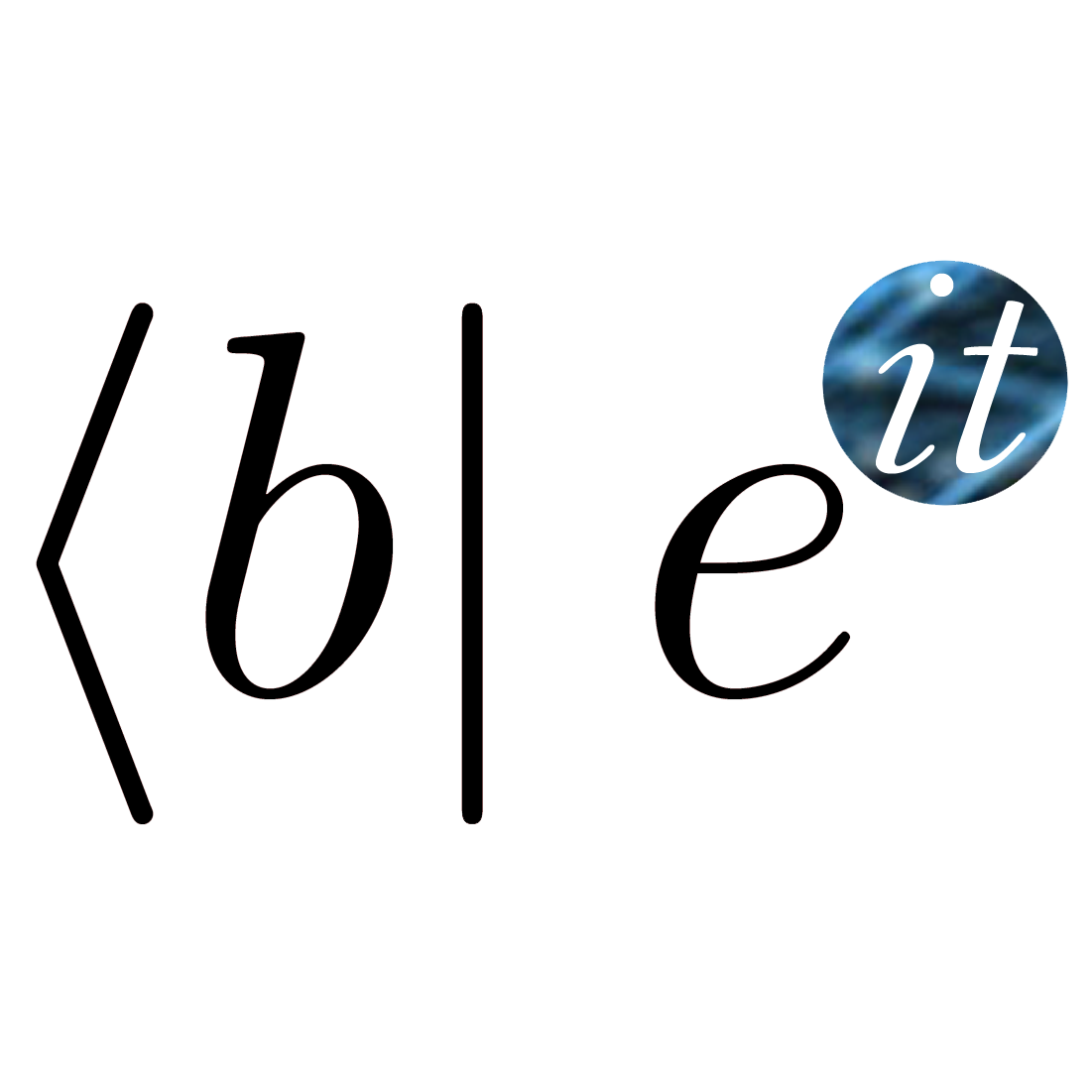On the hyperbolic Bloch transform
Ákos Nagy
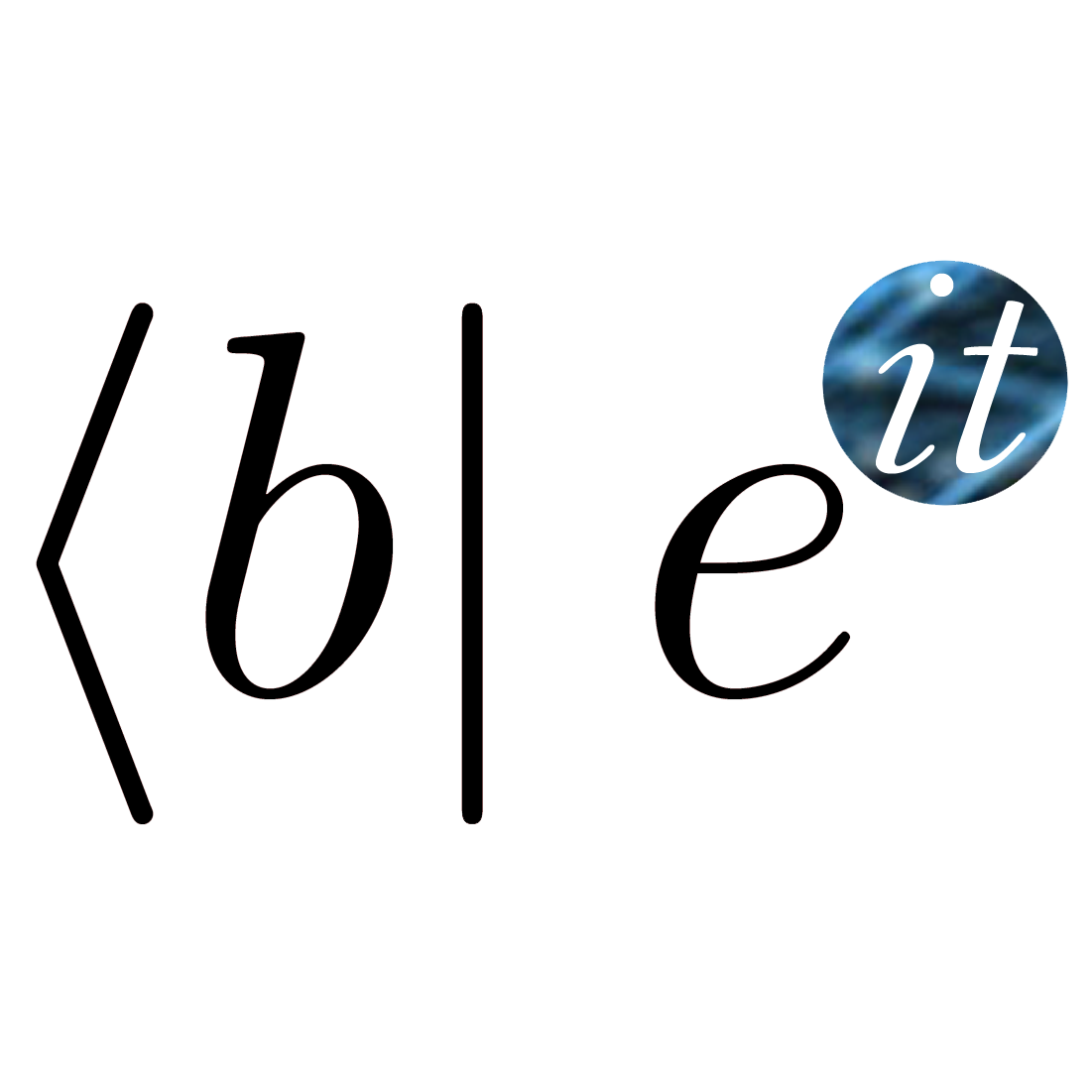
This talk is based on 2208.02749, (Annales Henri Poincaré, 2024).
Hyperbolic materials
- Recent development in condensed matter physics: realizations of periodic systems with effective theories on the hyperbolic plane.
- More precisely: translation invariant $\{ p, q \}$ tessellations with nearest neighbor interactions.
- The symmetry group is then a Fuchsian group
\begin{equation}
\Gamma = \left\langle \alpha_1, \beta_1, \ldots, \alpha_g, \beta_g \middle| \left[ \alpha_1, \beta_1 \right] \cdots \left[ \alpha_g, \beta_g \right] = \mathbb{I} \right\rangle \quad (4g = p).
\end{equation}
- Effective model: Hilbert space $\mathcal{H} \cong L^2 \left( \Gamma \right) \otimes \mathcal{H}_0$, with a Hamiltonian, $H$, that is $\Gamma$-periodic:
\begin{equation}
\Gamma \hookrightarrow \mathrm{Aut} \left( H \right) \cap \mathrm{U} \left( \mathcal{H} \right).
\end{equation}
- In classical crystallography ($\Gamma \cong \mathbb{Z}^{\dim}$) one studies the system $\left( \mathcal{H}, H \right)$ via the Bloch transform.
Warmup: The classical Bloch transform on the Euclidean plane, part 1.
- Let $\mathcal{H} = L^2 (\mathbb{R}^2)$ and $\Gamma = \mathbb{Z} \: v_1 \oplus \mathbb{Z} \: v_2 \cong \mathbb{Z}^2$.
- Let the Brillouin zone be $\mathrm{BZ} = \mathrm{Hom} \left( \Gamma, \mathrm{U} (1) \right) \cong$ torus.
- Each $\lambda \in \mathrm{BZ}$ gives a flat unitary connection, $\nabla^\lambda$, on $E = \mathbb{R}^2 / \Gamma \times \mathbb{C}$, unique up to gauge.
- Let $U_{\nabla^\lambda} (x) = e^{i k_\lambda \cdot x}$ be the lift of the holonomy of $\nabla^\lambda$ from $(0,0)$ to $x \in \mathbb{R}^2$.
- If $\psi \in \mathcal{H}$ and $y = \{ x_0 + R | R \in \Gamma \} \in \mathbb{R}^2 / \Gamma$, then let
\begin{equation}
\widetilde{\mathcal{B}} \left( \psi, \nabla^\lambda \right) \left( y \right) = \sum\limits_{x \in y} \psi (x) U_{\nabla^\lambda} (x) \in \mathrm{End} \left( E_y \right).
\end{equation}
- Note: $\lambda \left( R \right) = e^{2 \pi i k_\lambda \cdot R}$, where $[k_\lambda] \in \left( \mathbb{R}^2 \right)^* / \Gamma^*$.
Warmup: The classical Bloch transform on the Euclidean plane, part 2.
\begin{equation}
\mathcal{B} \left( \psi, \lambda \right) = \left[ \lambda, y \mapsto \sum\limits_{x \in y} \psi (x) U_{\nabla^\lambda} (x) \right] \in \left( \mathrm{BZ} \times L^2 \left( \mathrm{End} \left( E \right) \right) \right) / \mathrm{U} (1) = : L^2 \left( \mathcal{E} \right).
\end{equation}
- Now $\mathcal{B} \left( \psi \right)$ is an $L^2$ section of $\mathcal{E}$, a (trivial) $\mathrm{End} \left( E \right)$-bundle over $\mathrm{BZ}$, with some nice properties.
- Plancherel theorem: $\mathcal{B}$ is a unitary isomorphism.
- If $A : \mathcal{H} \rightarrow \mathcal{H}$ is a $\Gamma$-periodic operator, then $\widehat{A} = \mathcal{B} \circ A \circ \mathcal{B}^{- 1}$ is algebraic.
- In particular: $\mathcal{B} \left( \left( \Delta + V \right) \psi \right) = \left( \left( \nabla^\lambda \right)^* \nabla^\lambda + V \right) \mathcal{B} \left( \psi, \lambda \right)$.
- Interpretation: $\mathcal{B} \left( T_R \left( \psi \right), \lambda \right) = \lambda \left( R \right) \mathcal{B} \left( \psi, \lambda \right) \: \Rightarrow \: \mathcal{B}$ decomposes $\psi$ into quasi-periodic waves.
Warmup: The classical Bloch transform on the Euclidean plane, part 3.
- (Non)commutative geometric point of view: $C_\Gamma =$ group-$C^*$-algebra of $\Gamma$ (a Neumann algebra).
- $\mathcal{H}$ is a projective $C_\Gamma$-module.
- $C_\Gamma \cong C^0 \left( \mathrm{torus} \right)$.
- $C^0$-projective modules are vector bundles, $C_\Gamma$ periodic operators (elements of another Neumann algebra) are endomorphism of that bundle, etc...
- This approach is more natural, but less geometric/analytic.
Hyperbolic lattices
- Let $\mathcal{H} = L^2 \left( \mathbb{H} \right)$ and $\Gamma \subset \mathrm{Isom} \left( \mathbb{H} \right)$ be a Fuchsian group (of genus $g$).
- $\mathcal{H}$ is again a projective $C_\Gamma$-module.
- Problem: $C_\Gamma$ is a noncommutative space!
- Maciejko–Rayan: studied the rank-1 case of the Bloch transform.
- Rank-1 representation are insensitive to $\left[ \Gamma, \Gamma \right]$.
- In other words, any "geometrization" needs to use higher rank representations.
Hyperbolic Bloch transform through stable bundles, part 1.
- Let $\mathcal{H} = L^2 \left( \mathbb{H} \right)$ and $\Gamma \subset \mathrm{Isom} \left( \mathbb{H} \right)$ be a Fuchsian group (of genus $g$).
- One Brillouin zone for each rank $\: \mathrm{BZ}_\Gamma = \bigcup\limits_{n \in \mathbb{Z}_+}^\infty \overbrace{\mathrm{Hom}_{\mathrm{irr}} \left( \Gamma, \mathrm{U} (n) \right) / \mathrm{U} (n)}^{\mathrm{BZ}_\Gamma^n}$.
- Each $\varrho : \Gamma \rightarrow \mathrm{U} (n)$ irreducible representation determines an irreducible flat, Hermitian connection, $\nabla^\varrho$, on $E^n = \Sigma \times \mathbb{C}^n$, where $\Sigma = \mathbb{H} / \Gamma$, unique up to gauge. These are also the stable bundles of rank $n$ and degree $0$ (Narasimhan–Seshadri, Donaldson).
- Let $U_{\nabla^\varrho} : \mathbb{H} \rightarrow \mathrm{U} (n)$ as before; the lift of the holonomy of $\nabla^\varrho$ from $(0,0)$ to $x \in \mathbb{R}^2$.
- If $\psi \in \mathcal{H}$ and $y \in \Sigma$, then let
\begin{equation}
\widetilde{\mathcal{B}} \left( \psi, \nabla^\varrho \right) \left( y \right) = \sum\limits_{x \in y} \psi (x) U_{\nabla^\varrho} (x) \in \mathrm{End} \left( E_y^n \right).
\end{equation}
Hyperbolic Bloch transform through stable bundles, part 2.
\begin{equation}
\mathcal{B}_n \left( \psi, \varrho \right) = \left[ \varrho, y \mapsto \sum\limits_{x \in y} \psi (x) U_{\nabla^\varrho} (x) \right] \in \left( \mathrm{Hom}_{\mathrm{irr}} \left( \Gamma, \mathrm{U} (n) \right) \times L^2 \left( \mathrm{End} \left( E^n \right) \right) \right) / \mathrm{U} (n) = : L^2 \left( \mathcal{E}_\Gamma^n \right).
\end{equation}
- Now $\mathcal{B}_n \left( \psi \right)$ is an $L^2$ section of $\mathcal{E}^n$, a (trivial) $\mathrm{End} \left( E^n \right)$-bundle over $\mathrm{BZ}_\Gamma^n$, with some nice properties.
- If $A : \mathcal{H} \rightarrow \mathcal{H}$ is a $\Gamma$-periodic operator, then there are algebraic operators, $\widehat{A}_n$, such that
\begin{equation}
\mathcal{B} \circ A = \widehat{A}_n \circ \mathcal{B}.
\end{equation}
- In particular: $\mathcal{B} \circ \left( \Delta + V \right) = \left( \left( \nabla^\varrho \right)^* \nabla^\varrho + V \right) \circ \mathcal{B}$.
- Interpretation remains: $\mathcal{B} \left( T_\gamma \left( \psi \right), \varrho \right) = \varrho \left( \gamma \right) \mathcal{B} \left( \psi, \varrho \right) \: \Rightarrow \: \mathcal{B}$ decomposes $\psi$ into quasi-periodic waves.
- Missing: Plancherel theorem!
Main result
- Main Theorem; informal version (N–Rayan)
For each $\psi \in C_{\mathrm{cpt}}^0 \left( \mathbb{H} \right)$, the sequence $\left( \mathcal{B}_n^* \mathcal{B}_n \left( \psi \right) \right)_{n \in \mathbb{Z}_+}$, converges to $\psi$ in the topology of $L^\infty \left( \mathbb{H} \right)$.
- Proof uses a recent result of Magee:
\begin{equation}
\mathbb{E}_n \left( \gamma \right) = \int\limits_{\mathrm{BZ}_\Gamma^n} \mathrm{tr} \left( \varrho \left( \gamma \right) \right) \mathrm{d} \mu_n = \delta_{\mathbb{I}, \gamma} + O \left( \tfrac{1}{n} \right).
\end{equation}
- \begin{equation}
\left\langle \mathcal{B}_n^* \mathcal{B}_n \left( \psi \right) \middle| \psi^\prime \right\rangle - \left\langle \psi \middle| \psi^\prime \right\rangle = \sum\limits_{\gamma_1, \gamma_2 \in \Gamma} \left( \mathbb{E}_n \left( \gamma_1^{- 1} \gamma_2 \right) - \delta_{\gamma_1^{- 1} \gamma_2, e} \right) \int\limits_C \overline{\psi \left( \gamma_1^{- 1} (x) \right)} \psi \left( \gamma_2^{- 1} (x) \right) \mathrm{dA}_{\mathbb{H}} (x).
\end{equation}
- A stronger version of Magee's result can sharpen our theorem too.
Future wish list
- Plancherel theorem for the hyperbolic Bloch transform via strengthening Magee's theorem.
- Study spectral properties of the Laplaians of stable bundles.
- Construction of topological phases.
- Understanding connections to the Hitchin moduli space.
- Understanding connections to the Helgason's hyperbolic Fourier transform.
Thank you for your attention!